Servicios Personalizados
Articulo
Latin American applied research
versión On-line ISSN 1851-8796
Lat. Am. appl. res. vol.45 no.1 Bahía Blanca ene. 2015
Sorption isotherms and thermodynamic analysis of seed fruits used to obtain vegetable oil
K.S. Silva, J.T. Romero and M.A. Mauro
Department of Food Engineering and Technology, Institute of Biosciences, Language and Physical Sciences (IBILCE), UNESP - São Paulo State University Rua Cristóvão Colombo 2265, 15054-000 São José do Rio Preto, SP, Brazil. e-mail: keilasouzas@yahoo.com.br
Abstract— Sorption isotherms of papaya, melon and grape seeds were determined and thermodynamic properties compared. The experiments were carried out at different temperature using the gravimetric method. The Henderson and GAB models were the best which represented the experimental data. Papaya and grape seeds are more stable with larger moisture content than melon seeds at 30°C. Grape seeds presented more resistance to lose water during the dehydration. The differential enthalpy and entropy decreased with increasing moisture content and satisfied the compensation theory. It was found that the sorption process investigated was enthalpy-driven.
Keywords— Seeds; Grape Seeds; Melon Seeds; Papaya Seeds; Thermodynamics.
I. INTRODUCTION
Much of the fruit production is destined for processing candies, extracts, juices and pulps. Each processing, tonels of waste are generated and discarded, causing problems to the environment and to the companies. Some studies emphasize the use of seeds from industrial discharges to obtain vegetable oils (Arvanitoyannis et al., 2006; Jorge and Malacrida, 2008; Jorge et al., 2009). Besides being consumed in the food, this vegetable oil can be used in the chemical industry, pharmaceutical and biodiesel production. Malacrida (2009) noted high lipid content in Papaya seeds (Carica papaya L.) and melon seeds (Cucumis melo inodorus) when compared to the content found in soybean seeds and indicated the studied seeds as a good source of oil. The study showed that the papaya and melon seeds presented higher protein content than that found in rice, corn, oats and wheat. According to Crews et al. (2006), grape seed oil has a high content of unsaturated fatty acids and phytosterols, suitable for a diet to reduce cholesterol levels. For a large scale production is necessary to know some characteristics of seeds and their stability attributes, as well as the process variables, because to obtain vegetable oil is necessary that the seeds are dehydrated. The knowledgement of the existent relations between the temperature and the air relative moisture is very important to control microbial growth and ensure seed quality during storage, because these tend to exchange moisture with the atmosphere surrounding them. This exchange of moisture content can be increased or reduced according to the hygroscopicity of the seeds and the gradient of potential hidric between the air atmospheric and the seeds (Rizvi, 1986).
The relationship between the water activity and equilibrium moisture content of a product subject to a specific temperature are expressed graphically by the curves of sorption isotherms (Rizvi, 1986). These curves are obtained at constant temperature and pressure and vary with the physical and chemical structure of food (Chirife and Iglesias, 1978). When the product is a solid, the water interactions occur not only in aqueous solution, but also depend on factors such as capillarity and hydration forces, responsible for binding more strongly the water to insoluble macromolecules of food. For this reason the relation between the equilibrium moisture and the water activity in solid foods is usually determined experimentally for each product. From the graphs of sorption isotherms point it is possible to determine thermodynamic functions such as enthalpy differential, which provides informations on the state where water is present in a biological material (Fasina et al., 1997) and the differential entropy, which informs the number of sorption sites for a given level of energy inherent in biological materials (Madamba et al., 1996). This manner it is possible to predict the required energy consumption for the dehydration process as well as the more stable conditions of seeds to the storage.
According to Ferro-Fontan et al. (1982), some foods present a linear correlation between the enthalpy and entropy for water sorption. This linearity is represented by the compensation theory and confirmed by several researchers (Madamba et al., 1996; Telis et al., 2000; McMinn et al., 2005; Fasina, 2006). The slope of the straight represents the temperature at which all reactions in series proceed at the same rate: the isokinetic temperature (TB).
This work aimed to determine and model the sorption isotherms of papaya seeds, melon seeds and grape seeds using several mathematical models, to determine and compare some thermodynamic properties of the studied seeds and to evaluate the application of the enthalpy-entropy compensation theory.
II. MATERIALS AND METHODS
A. Materials
Sorption isotherms of seeds of grape (Vitis vinifera), papaya (Carica papaya L.) and melon (Cucumis melo inodorus) were accomplished. The seeds were maintained in cold chamber (5°C) until the experiment. Saturated aqueous salt solutions were prepared using NaOH, LiCl, KC2H3O2, MgCl2, K2CO3, Mg(NO3)2, NaNO2, NaCl, KCl, BaCl2 and CuSO4 (all analytical grade) and commercial formaldehyde (40%) was used for cleaning the material and preventing microbial spoilage of samples.
B. Samples
The seeds were separated from fruits waste by hand, washed quickly with distilled water and dried in tray at room temperature. The seeds were homogenized and destined to the experiment.
C. Sorption isotherms
Sorption isotherms were plotted for the seeds, based on static gravimetrical methods proposed by Jowitt et al. (1987). Ten saturated aqueous salt solutions were prepared corresponded to water activity intervals between 0.02 to 0.97. Duplicate samples were weighed into small plastic receptacles and placed on tripods in the jars, which were then tightly closed and placed in a temperature-controlled chamber. The interval of studied temperature was from 30°C to 70° C. The required equilibration time was 4 to 5 weeks based on the change in weight expressed on a dry basis, which did not exceed 0.1% (0.001g/g dry solids). The equilibrium moisture content was measured gravimetrically in triplicate, drying to constant weight using a vacuum oven at 60°C.
D. Data analysis
Sorption Isotherms
For the mathematical description of sorption isotherms were adjusted four mathematical models to the experimental data (Table 1) using the non-linear regression module of Statistica 7.0 software (Statsoft, Tulsa, OK, USA). Following the methodology proposed by Peleg (1993), regressions were repeated with various initial estimated values both above and below those that had been calculated to confirm whether or not convergence were reliable.
Table 1: Mathematical models applied to the experimental sorption data.
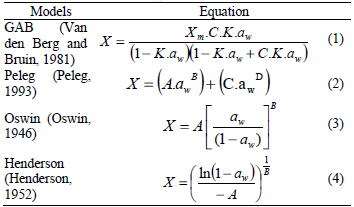
where aw is water activity, dimensionless; X is equilibrium moisture (% dry basis); Xm is moisture content of monolayer (% dry basis); A, B, C, D and K are parameters of isotherm equations.
The goodness of fit of the different models was evaluated with the regression coefficient (R2) and the mean relative deviation modulus (Me). Based on Eq. (5), Lomauro et al. (1985) considered mean relative percent deviation values below 10% a reasonable adjustment for the proposal practices.
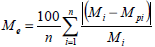 | (5) |
where Mi is experimental moisture content values (kg water kg-1 dry solids), Mpi is moisture content values predicted by the model (kg water kg-1 dry solids) and n is number of experimental data.
Thermodynamic properties
The differential enthalpy of water sorption (Δh) or net isosteric heat of sorption, is defined as the difference between the total differential enthalpy of sorption (ΔH) point it and the molar enthalpy of vaporization of pure water (kJ/mol) (ΔHvap) and can be determined from Eq. (6), which is derived from the Clausius - Clapeyron equation, applied to food and pure water (Rizvi, 1986).
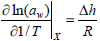 | (6) |
where R is the gas constant (8.314 J.mol-1.K-1), aw the water activity and T the temperature (K).
Assuming that the sorption differential enthalpy be independent of temperature changeness, ln(aw) was plotted versus 1/T for a specific moisture content of the material and determined the slope of the curve, which equals (Δh/R) and the linear coefficient of the curve (Δs/R) where Δs is the differential entropy (J.mol-1.K-1) (Tsami et al., 1990; McMinn and Magee, 2003). The model that best fitted the experimental data was used to determine the value for each moisture content in order to determine the dependence of aw on the moisture content (X), at each temperature. This way, the differential enthalpy and entropy of sorption can be determined from moisture sorption data by using the Eq. 7 (Fasina et al., 1997):
 | (7) |
According to Ferro-Fontan et al. (1982), some foods present a linear relationship between the enthalpy and entropy for water sorption. This linear relationship is represented by compensation theory (Eq. 8) and confirmed by several other authors (Madamba et al., 1996; Telis et al., 2000; McMinn et al., 2005; Fasina, 2006). The slope of the straight represents the temperature at which all reactions in series proceed at the same rate: the isokinetic temperature (TB).
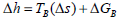 | (8) |
where ΔGB is the free energy at TB.
The isokinetic temperature was compared with harmonic mean temperature to confirm the compensation theory, as recommended by Krug et al. (1976). According to the author, if the temperatures are different, the compensation theory can be applied for the studied product. The Eq. 9 defines the harmonic mean temperature, where n represents the temperatures number used.
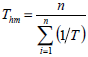 | (9) |
The Eq. 10 expresses an approximate (1-α)100% percent confidence interval for isokinetic temperature, where TB and Var(TB) are calculated according to Eqs. 11 and 12, respectively.
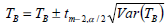 | (10) |
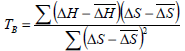 | (11) |
 | (12) |
where m is the number of data pairs of enthalpy and entropy; 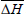 is the mean differential enthalpy (J.mol-1);
is the mean differential enthalpy (J.mol-1); 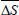 is the mean differential entropy (J.mol-1) and
is the mean differential entropy (J.mol-1) and 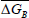 is the Gibbs free energy at isokinetic temperature (J.mol-1).
is the Gibbs free energy at isokinetic temperature (J.mol-1).
III. RESULTS AND DISCUSSION
The sorption isotherms of papaya, melon and grape seeds obtained experimentally are presented in the Fig. 1, 2 and 3, respectively.

Fig. 1. Experimental values of equilibrium moisture content (dry basis) as a function of water activity for papaya seeds at different temperatures.
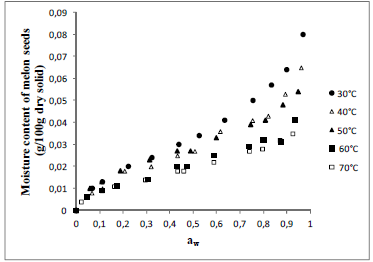
Fig. 2. Experimental values of equilibrium moisture content (dry basis) as a function of water activity for melon seeds at different temperatures.
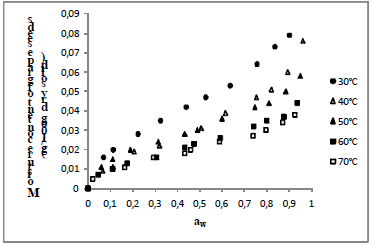
Fig. 3. Experimental values of equilibrium moisture content (dry basis) as a function of water activity for grape seeds at different temperatures.
The isotherms of all seeds showed the typical sigmoid shape of Type II, according to the BET classification, describing the seeds as no porous or macroporous materials with low sorption energy (Condon, 2006). The same format was observed for quinoa seeds (Miranda et al., 2012), pumpkin seeds (Mayor et al., 2005), pea seeds (Chen, 2003) and lentil seeds (Nikolay, 2000).
Observe that the effect of temperature in sorption isotherms is very pronounced and as the temperature increases occurs a fall in the equilibrium moisture content of seeds, resulting in less hygroscopic product. This behaviour, generally, ascribed to a reduction in the number of active sites, due to the physical and chemical changeness induced by temperature (Rizvi, 1986). The reduction of these sites would result less energy for the interaction with the water molecules resulting in less hygroscopic seeds (Ascheri et al., 2009).
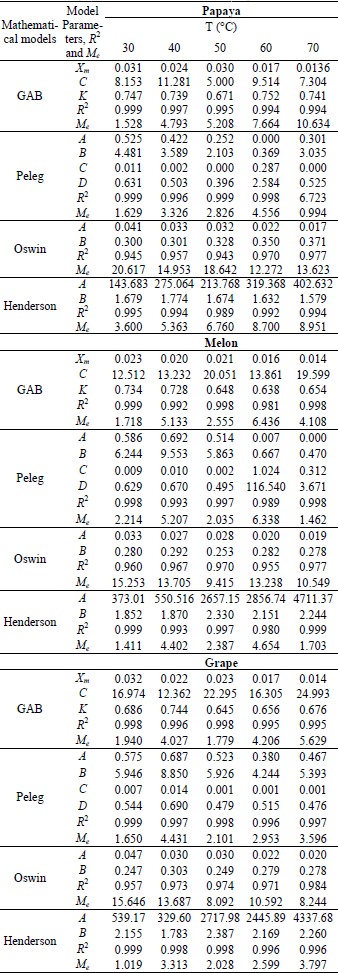
For the grape and melon seeds, the Henderson model presented the best fit to the experimental data showing higher values of regression coefficient (R2) and smaller relative percent deviation (Me) (below of 10%), already for papaya seeds, the GAB model presented the best fit to the experimental data. The results of nonlinear regression analysis of experimental data, (R2) values and the mean relative percent deviation values (Me) are presented in Table 2.
Table 2: Estimated values of coefficients, regression coefficient (R2) and relative percent deviation (Me) obtained for selected sorption models applied to experimental sorption data for papaya seed, melon seed and grape seed.
Mayor et al. (2005) noted GAB, Peleg and Henderson models were the best fit the experimental data for pumpkin seeds. For tomato seeds, Sogi et al. (2003) observed that Henderson model was the best fit to the experimental data. Francisco et al. (2007), although, noted Oswin and Peleg models were the best fit for Seed of two bean cultivars. The Peleg model, for presenting four parameters, almost ever result in an excellent fit to the sorption isotherms data, thus the resultant parameters of this model are empirical.
The GAB model is much used to foods because it presents physical meaning. The moisture content corresponding to an adsorbed monolayer (Xm), provided by this model has a remarkable importance in food storage and deterioration study, because represents the quantity of water that is strongly bounded to the actives sites and aid to define the chemical and physical stability.
Observe in Table 2 the moisture of monolayer obtained for each seed tend to reduce with temperature. The increase in temperature provides an increase on disorder of water molecules resulting in the breaking of the intermolecular bounds between water molecules and the sorption sites present in seeds and following decreasing the monolayer moisture content (Hossain et al., 2001).
The papaya and grape seeds showed bigger and similar monolayer moisture (about de 3.1g/100g dry solid) than melon seeds at 30°C (2.34g/100g dry solid), indicating that the first seeds hold bigger stability at high moisture (Table 2). However, from 60°C was not observed more difference between monolayer moisture of seeds, probably due to chemical and physical changeness provided by temperature. According to Vishwakarma et al. (2011), a product with moisture content equal to the moisture of monolayer can be storage for long periods with a minimum quality loss. Mayor et al. (2005) observed moisture monolayer values for pumpkin seeds at 25°C around 5.65g/100g dry solid.
To determine the value of aw for each equilibrium moisture content, was chosen the Henderson model, because it was the best fit for the majority seeds. The differential enthalpy (Δh) was calculated according to the Eq. 7. Due to the bigger value of differential enthalpy (values less negatives) (Fig. 4), note that grape seeds present bigger resistance to lose water and thus, requires a larger spend energetic for its dehydration. This occurs due to the water molecules in grape seeds being strongly bounded in the monolayer form.
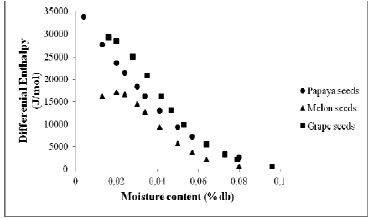
Fig. 4. Differential enthalpy of papaya seeds, melon seeds and grape seeds as a function of equilibrium moisture content.
The differential entropy values (Δs) were determined through linear regression using Eq. 7. The differential entropy in function of equilibrium moisture content is presented in Fig. 5. Observe a decreasing of differential entropy values with increasing in moisture of seeds that can be related.

Fig. 5. Differential entropy of papaya seeds, melon seeds and grape seeds as a function of equilibrium moisture content.
Melon seeds showed smaller differential entropy (values more negatives), indicating high mobility water molecules and, consequently, less difficulty for dehydration.
The entropy values correspondent to the monolayer values (Table 2) of papaya, melon and grape seeds were: 50.96; 44.73; 60.28 J/mol.K, respectively.
The correlation between Δh and Δs for the tree studied seeds, established through Eq. 8, introduced linear behaviour, indicating the existence of compensation theory for papaya, melon and grape seeds. Isokinetic temperature (TB), Gibbs free energy at isokinetic temperature (ΔGB) and R2 are showed in Table 3.
Table 3: Temperature isokinetic, Gibbs free energy at isokinetic temperature and regression coefficient obtained for papaya seeds, melon seeds and grape seeds.
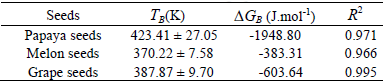
Harmonic mean temperature (Thm), calculated according to the Eq. 5, was 322.38K. According to Leffler (1955), the process is enthalpy-driven when TB >Thm and entropy-controlled when TB <Th. Note that all seeds showed TB >Thm indicating enthalpy-driven process (Table 3), in other words, water vapor sorption mechanisms of papaya, melon and grape seeds are controlled by energetic interaction and not by seeds microstructure. Thys et al. (2010) noted the same behaviour for Araucaria angustifolia seeds, thus isokinetic temperature calculated by authors (421 ± 17K) was very close to the ones which were obtained for papaya seeds.
The clarification about the hygroscopicity of the papaya, melon and grape seeds and its water vapor sorption mechanisms, obtained in this study, is very important to control microbial growth and to ensure seed quality during storage.
IV. CONCLUSIONS
Between studied models (Henderson, GAB, Peleg and Oswin), The Henderson model was the best to represent the experimental data obtained to construct of sorption isotherms of grape seeds and melon seeds. For papaya seeds, though, the GAB model was the best fit to experimental data. Papaya and grape seeds are stabler with larger moisture content than melon seeds at 30°C. Grape seeds present bigger enthalpy differential value and bigger differential entropy value, indicating, consequently, more resistance to lose water during dehydration of product. Plots of differential enthalpy versus differential entropy satisfied the enthalpy-entropy compensation theory (isokinetic theory). The results suggest that the sorption process of papaya seeds, melon seeds and grape seeds are enthalpy-driven.
REFERENCES
1. Arvanitoyanni, I.S., S.D. Ladas and A. Mavromatis, "Potential uses and applications of treated wine waste: a review," International Journal of Food Science and Technology, 41, 475-487 (2006).
2. Ascheri, D.P.R., W.S. Moura, J.L.R. Ascheri and E.A.F. Junior, "Propriedades termodinâmicas de adsorção de água do amido rizomas do lírio-do-brejo (Hedychium coronarium)," Food Science and Technology, 29, 454-462 (2009).
3. Chen, C., "Moisture sorption isotherms of pea seeds," Journal of Food Engineering, 58, 45-51 (2003).
4. Chirife, J. and H.A. Iglesias, "Equations for fitting water sorption isotherms of foods Part I. A review," Journal of Food Technology, 13, 159-174 (1978).
5. Condon, J.B., Surface area and porosity determinations by physisorption. Measurements and theory, Elsevier, Amsterdam, UK (2006).
6. Crews, C., P. Hough, J. Godward, P. Brereton, M. Lees, S. Guiet and W. Winkelmann, "Quantitation of the main constituents of some authentic grape-seed oils of different origin," Journal of Agricultural and Food Chemistry, 54, 6261-6265 (2006).
7. Fasina, O., S. Sokhansanj and R. Tyler, "Thermodynamics of moisture sorption in alfalfa pellets," Drying Technology, 15, 1553-1570 (1997).
8. Fasina, O., "Thermodynamic properties of sweet potato," Journal of Food Engineering, 75, 149-155 (2006).
9. Ferro-Fontan, C., J. Chirife, E. Sancho and H.A. Iglesias, "Analysis of a model for water sorption phenomena in foods," Journal of Food Science, 55, 475-477 (1982).
10. Francisco, F.G., R. Usberti and J.T.C.L. Toneli, "Ajuste de isotermas de sorção de sementes de cultivares de feijoeiro," Revista Brasileira de Sementes, 29, 35-39 (2007).
11. Henderson, S.M., "A basic concept of equilibrium moisture," Agricultural Engineering, 33, 29-32 (1952).
12. Hossain, M.D., B.K. Bala, M.A. Hossain and M.R.A. Mondol, "Sorption isotherms and heat of sorption of pineapple," Journal of Food Engineering, 48, 103-107 (2001).
13. Jorge, N. and C.R. Malacrida, "Extratos de sementes de mamão (Carica Papaya L.) como fonte de antioxidantes naturais," Alim. Nutr., 19, 337-340 (2008).
14. Jorge, N., C.R. Malacrida, P.M. Angelo and D. Andreo, "Composição centesimal e atividade antioxidante do extrato de sementes de maracujá (Passiflora edulis) em óleo de soja," Pesq. Agropec. Trop., 39, 380-385 (2009).
15. Jowitt, R., F. Escher, B. Hallstom, H.F.T. Meffert, W.E.L. Spiess and G. Vos, Physical Properties of Foods, Applied Science Publishers. London and New York (1987).
16. Krug, R.R., W.G. Hunter and R.A. Grieger, "Enthalpy-entropy compensation. 1- Some fundamental statistical problems associated with the analysis of Van't Hoff and Arrhenius data." Journal of Physical Chemistry, 80, 2335-2341 (1976).
17. Leffler, J.E., "The Enthalpy-entropy relationship and its implications for organic chemistry," J. Organic Chemistry, 20, 202-1231 (1955).
18. Lomauro, C.J., A.S. Bakshi and T.P. Labuza, "Evaluation of food moisture sorption isotherm equations. Part 1. Fruit, vegetable and meat products," Lebensmittel-Wissenschaff und Tecnolgie, 18, 111-117 (1985).
19. Madamba, P.S., R.H. Driscoll and K.A. Buckle, "Enthalpy-entropy compensation models for sorption and browning of garlic," Journal of Food Engineering, 28, 109-119 (1996).
20. Malacrida, R.C., Caracterização de óleos extraídos de sementes de frutas: Composição de ácidos graxos, tocoferóis e carotenóides, Master Thesis. Institute of Biosciences, Language, and Physical Sciences (IBILCE), Paulista State University (UNESP), Brazil (2009).
21. Mayor, L., R. Moreira, F. Chenlo and A.M. Sereno, "Water sorption isotherms of fresh and partially osmotic dehydrated pumpkin parenchyma and seeds at several temperatures," Eur. Food Res. Technol., 220, 163-167 (2005).
22. McMinn, W.A.M. and T.R.A. Magee, "Thermodynamic properties of moisture sorption of potato," Journal of Food Engineering, 60, 157-165 (2003).
23. McMinn, W.A.M., A.H. Al-Muhtaseb and T.R.A. Magge, "Enthalpy-entropy compensation in sorption phenomena of starch materials," Food Research International, 35, 505-510 (2005).
24. Miranda, M., A. Vega-Gálvez, M. Sanders, J. López, R. Lemus-Mondaca, E. Martínez and K. Di Scala, "Modelling the water sorption isotherms of quinoa seeds (Chenopodium quinoa Willd.) and determination of sorption heats," Food Bioprocess Technology. 5, 1686-1693 (2012).
25. Nikolay, D.M., "Moisture sorption isotherms of lentil seeds at several temperatures," Journal of Food Engineering, 44, 205-211 (2000).
26. Oswin, C.R., "The kinetics of package life III. The isotherm," Journal of Chemical Industry, 65, 419-421 (1946).
27. Peleg, M., Assessment of a semi-empirical four parameter general model for sigmoid moisture sorption isotherms, Journal of Food Process Engineering, 16, 21-37 (1993).
28. Rizvi, S.S.H., "Thermodynamic properties of food in dehydration," Engineering Properties of Foods (M.A. Rao and S.S.H. Rizvi, eds), 133-214 (1986).
29. Sogi, D.S., U.S. Shivhare, S.K. Garg and A.S. Bawa, "Water Sorption Isotherm and Drying Characteristics of Tomato Seeds," Biosystems Engineering, 84, 297-301 (2003).
30. Telis, V.R.N., A.L. Gabas, F.C. Menegalli and J. Telis-Romero, "Water sorption thermodynamic properties applied to persimmon skin and pulp," Thermochimica Acta, 343, 49-56 (2000).
31. Thys, R.C.S., C.P.Z. Noreña, D.F. Marczak, G.A. Aires and F. Cladera-Olivera, "Adsorption isotherms of pinhão (Araucaria angustifolia eeds) starch and thermodynamic analysis," Journal of Food Engineering, 100, 468-473 (2010).
32. Tsami, E., Z.B. Maroulis, D. Morunos-Kouris and G.D. Sarvacos, "Heat of sorption of water in dried fruits," International Journal of Food Science and Technology, 25, 350-359 (1990).
33. Van den Berg, C. and S. Bruin, "Water activity and its estimation in food systems," Water activity: influences on food quality (L.B. Rockland and G.F. Stewart, Eds.), New York: Academic Press, 147-177 (1981).
34. Vishwakarma, R.K., U.S. Shivhare and S.K. Nanda, "Moisture dsorption isotherms of guar (Cyamposis tetragonoloba) grain and guar gum splits," LWT - Food Science and Technology, 44, 969-975 (2011).
Received: November 19, 2012.
Accepted: February 12, 2013.
Recommended by Subject Editor: Mariano Martin Martin.